Data
- Title: Big data approach to Kazhdan–Lusztig polynomials
- Authors: Abel Lacabanne, Daniel Tubbenhauer and Pedro Vaz
- Status: preprint. Last update: Mon, 2 Dec 2024 08:55:44 UTC
- Code and (possibly empty) Erratum: Click
- Interactive calculation for \(S_{10}\): Click
- ArXiv link: https://arxiv.org/abs/2412.01283
Abstract
We investigate the structure of Kazhdan–Lusztig polynomials of the symmetric group by leveraging computational approaches from big data, including exploratory and topological data analysis, applied to the polynomials for symmetric groups of up to 11 strands.
A few extra words
The Kazhdan–Lusztig (KL) polynomials are fundamental yet enigmatic objects in combinatorial representation theory. First introduced
in the 1970s for arbitrary Coxeter groups, this paper focuses on the type A Coxeter group, the symmetric group
\(S_{n}\) on \(1,\dots,n\). In this context, KL polynomials represent entries in a nonnegatively graded change-of-basis matrix
between simple and Verma modules of \(\mathfrak{sl}_{n}\). As a result, these polynomials are either zero or belong to \(1+v\mathbb{Z}_{\geq 0}[v]\), where \(v\) denotes the grading variable.
Although a main focus of research in combinatorics, geometry, and representation theory alike, not much is known about these polynomials.
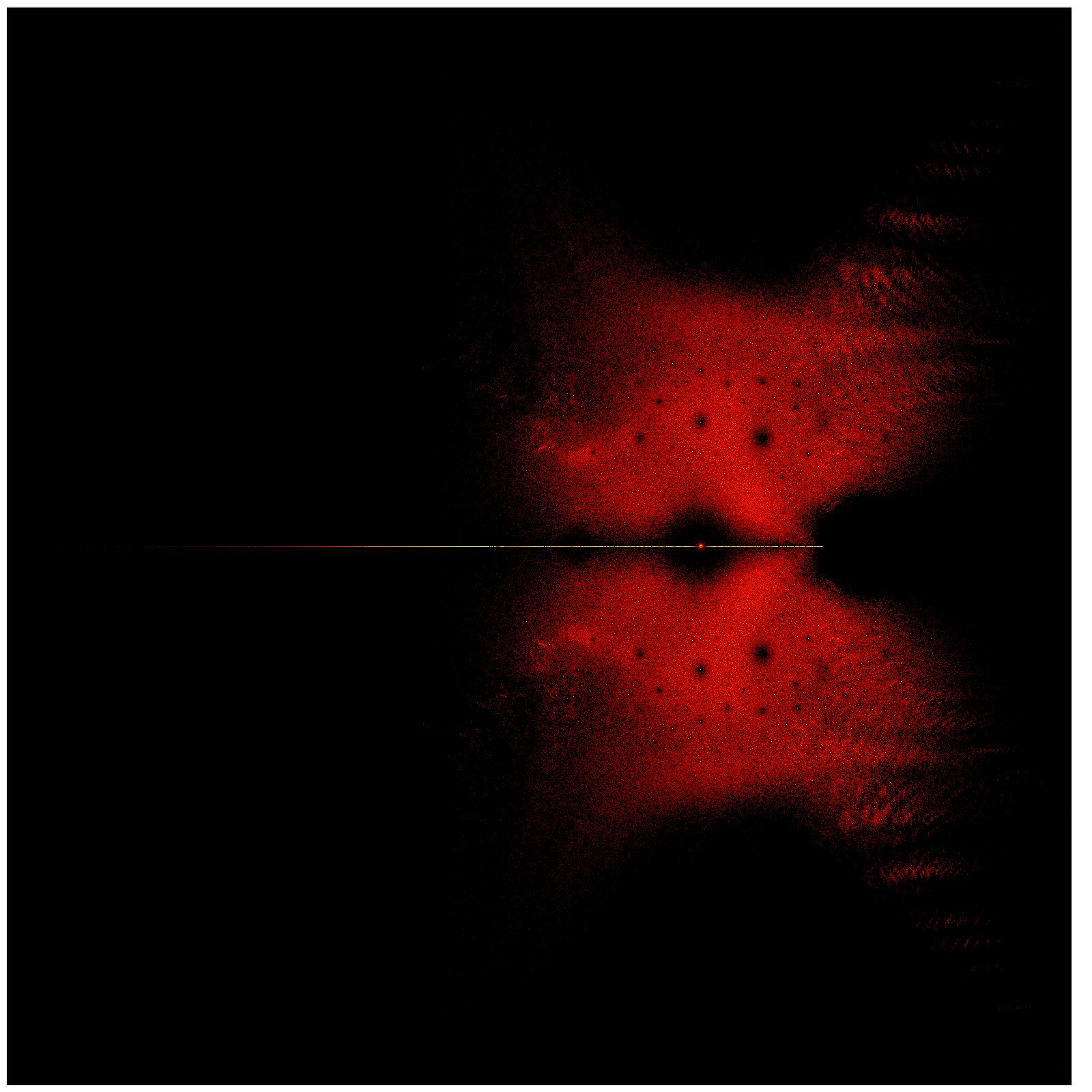
The starting point of this work is the observation that KL polynomials exhibit patterns akin to statistical distributions. By analyzing these distributions, we aim to uncover
structures that remain elusive through traditional methods, such as combinatorial or geometric approaches.
Our methodology involves systematic data processing and analysis, often referred to as ``big data,'' utilizing techniques such as data visualization, exploratory data analysis (EDA), and topological data analysis (TDA).
Using these methods, we will discuss several conjectures about KL polynomials and their distribution, and for some of them we give an indication of how to prove these conjectures.
For example, here is the distribution of their roots for \(n=11\):
