Data
- Title: Growth problems for representations of finite monoids
- Authors: David He and Daniel Tubbenhauer
- Status: To appear in North-West. Eur. J. Math. Last update: Wed, 5 Feb 2025 03:03:57 UTC
- Code and (possibly empty) Erratum: Click
- ArXiv link: https://arxiv.org/abs/2502.02849
Abstract
We give a conjecture for the asymptotic growth rate of the number of indecomposable summands in the tensor powers of representations of finite monoids, that it can be obtained from the (Brauer) character table of the monoid's group of units. We prove it under an additional hypothesis. We also give (exact and asymptotic) formulas for the growth rate of the length of the tensor powers when working over a good characteristic. As examples, we compute the growth rates for the full transformation monoid, the symmetric inverse monoid, and the monoid of 2 by 2 matrices. We also provide code used for our calculation.
A few extra words
Let \( M \) be a finite monoid, and let \( k \) be a splitting field for \( M \) of characteristic \( p \ge 0 \). For a \( kM \)-module \( V \), we are interested in the quantity \[ b(n) = b^{M,V}(n) = \text{the number of \( M \)-indecomposable summands in } V^{\otimes n} \text{ (counted with multiplicity)}. \] In particular, we ask the following questions:
- Can we find a formula for \( b(n) \), or more generally a formula for an asymptotic expression \( a(n) \) with \( b(n) \sim a(n) \)? (Here \( b(n) \sim a(n) \) means that \( \frac{b(n)}{a(n)} \xrightarrow{ n \to \infty } 1 \).)
- Can we understand the rate of geometric convergence by quantifying how fast \( | \frac{b(n)}{a(n)} - 1 | \) converges to 0?
- Similarly, can we bound the variance \( | b(n) - a(n) | \)?
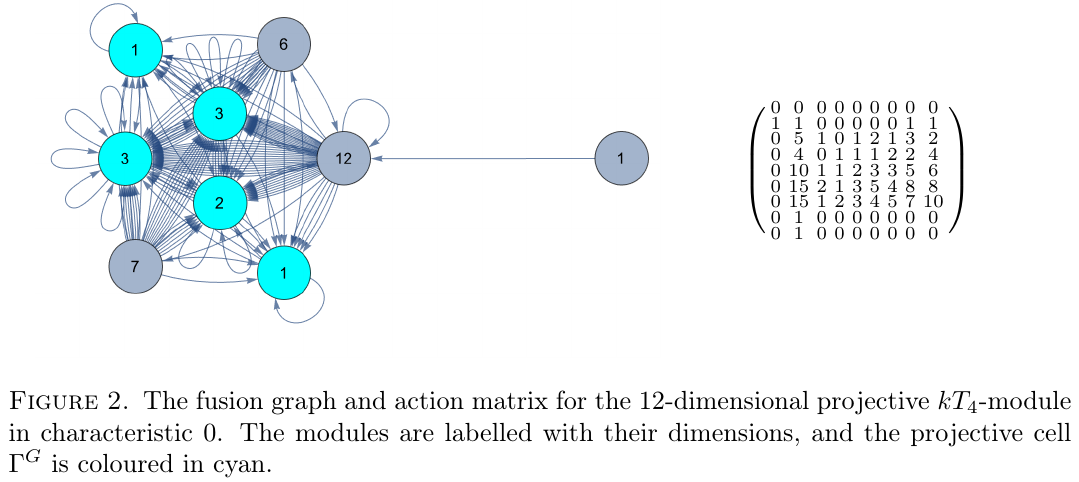
For example, we prove the conjecture under the hypothesis that some projective \( kG \)-module is injective as a \( kM \)-module. While the hypothesis is somewhat restrictive, it is satisfied by important classes of monoids such as the full transformation monoids \( T_n \) (in characteristic 0), the monoid of \( 2 \times 2 \) matrices \( M(2,q) \) (over defining characteristic), and all monoids with semisimple monoid algebra.