Data
- Title: Verma Howe duality and LKB representations
- Authors: Abel Lacabanne, Daniel Tubbenhauer and Pedro Vaz
- Status: To appear in New York J. Math. Last update: Fri, 9 Dec 2022 08:02:11 GMT
- ArXiv link: http://arxiv.org/abs/2207.09124
- LaTex Beamer presentation: Slides1
Abstract
We establish a version of Howe duality that involves a tensor product of Verma modules. Surprisingly, this duality leaves the realm of lowest and highest weight modules. We quantize this duality, and as an application, we prove that the (colored higher) LKB representations arise from this duality and use this description to show that they are simple as modules for the braid group and for various of its subgroups, including the pure braid group.
A few extra words
Arguably the most classical form of Howe duality relates commuting
actions of \(\mathrm{GL}_{m}(\mathbb{C})\) and \(\mathrm{GL}_{n}(\mathbb{C})\) on the symmetric
algebra of \(\mathbb{C}^{m}\otimes\mathbb{C}^{n}\).
Howe's approach turned out to be a game changer, even in fields
beyond representation theory. For example,
quantum versions of these dualities
provide powerful and categorification-friendly
descriptions of quantum invariants
such as the colored Jones polynomial.
In this paper we prove a version of the Howe duality above
where symmetric powers are replaced by Verma modules.
We also extend the
aforementioned application to quantum invariants:
we show that
the LKB (Lawrence-Krammer-Bigelow representations}
and their colored and higher counterparts arise from
this Verma Howe duality, which in turn enables us to
verify that the LKB representations are simple modules of various subgroups
of Artin's braid group.
In a few more details, the main theorem of this paper reads:
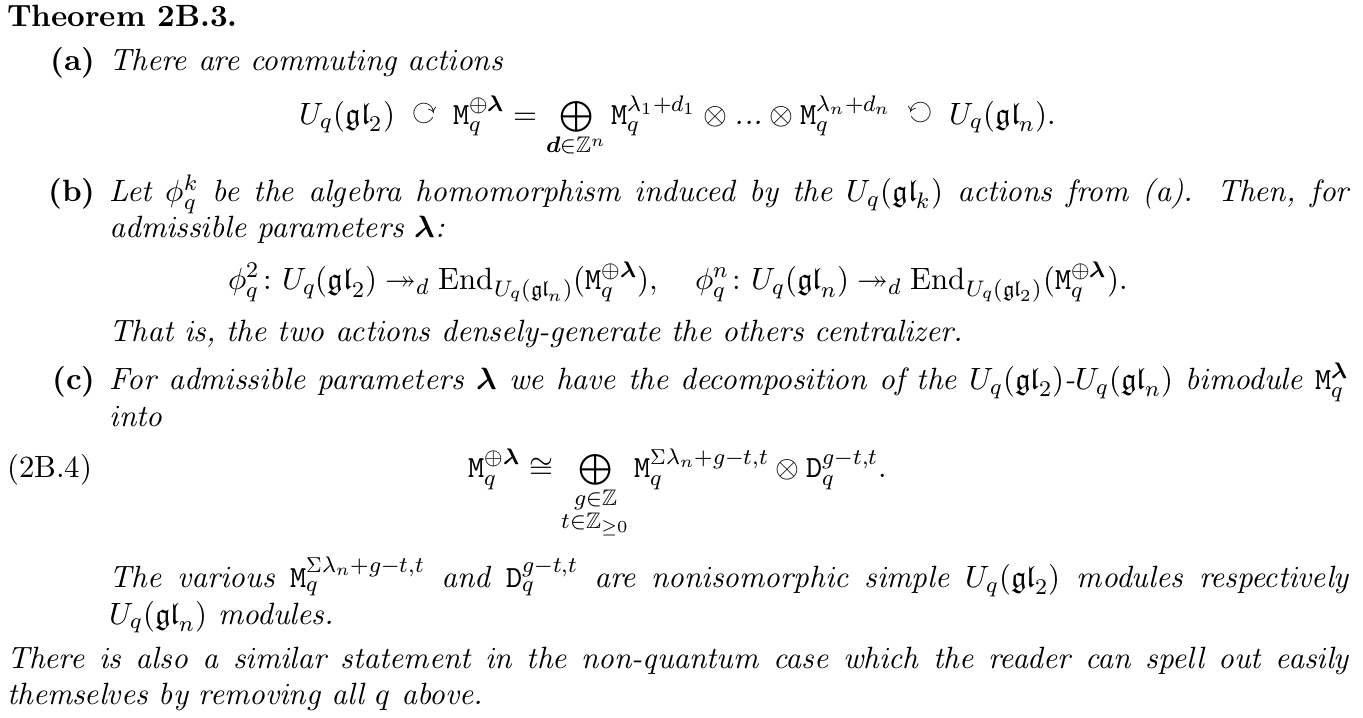
This can be seen as a Verma version of the classical Howe dualities, or even more fundamentally, of Schur-Weyl duality. For comparison, the latter roughly reads as